1 Introduction
Ball-end milling cutters are important tools for CNC machining complex surfaces (especially free-form surfaces), and the market demand is large. Due to the complicated blade shape of the ball-end milling cutter, most of the machining of the ball-end milling cutter at home and abroad needs to be realized on the multi-axis linkage CNC machine tool. Because the equipment is expensive (up to millions of dollars), the single-piece machining cost of the tool is higher. high. In order to reduce the cost of tool processing, the author began to study the non-CNC machining method of ball-end milling cutters in 1991, and together with the co-investigators, proposed the mathematical model of the rake face and the flank face of the ball-end milling cutter, and discussed the hard The related machining model of the ball-end milling cutter and the sequence production of the ball-end milling cutter. In the in-depth research and development of the ball-end milling cutter, in order to solve the complicated problem of the flank grinding mechanism in the original machining scheme, the plane curve master is used instead of the space curve master to process and succeed, further reducing the milling. Knife processing costs.
In order to make the general concept of the principle and method of non-CNC machining of ball-end milling cutters, this paper gives a summary introduction to the processing principles and related main mathematical models for final product formation.
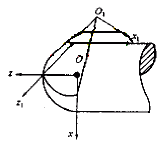Figure 1 Principle of rake face processing |
In order to facilitate cutting, the edge curve of the ball end mill should be an "S" spherical curve, that is, the intersection of the rake face and the spherical surface formed by the machining should be an "S" curve. The principle of the front face of the ball end mill designed for this purpose is shown in Fig. 1. Machining, conical wheel rotation about a fixed axis O
1, while the workpiece (workpiece mill) O
z rotation about its axis, enveloping surface contoured surface sand Group conical wheel relative to the workpiece is the movement of the rake face is formed.
In order to establish the main mathematical model of the rake face machining, the right-handed rectangular coordinate system s=[O;x,y,z] and s 1 =[O 1 ;x 1 ,y 1 , respectively, fixed on the workpiece and the grinding wheel are selected. z 1 ] (see Figure 1), where y and y1 are parallel and pointing upwards. If the radius of the big end of the grinding wheel is R 2 , the half apex angle of the cone wheel is g, the distance from the big end of the grinding wheel to O 1 is p, and the angle between the z and z 1 axes is f, then when the radius of the ball head is R, The transformation from the coordinate system s 1 to the coordinate system s is
| r ={x,y,z}={x 1 cosf+z 1 sinf-psinf,y 1 +R 2 ,x 1 sinf+z 1 cosf+R-pcosf} | (1) |
The contour equation of the grinding wheel in the coordinate system s
1 is
| r 1 ={x 1 ,y 1 ,z 1 }={ucosv,usinv,p+(R 2 -u)cotg} | (2) |
The contour of the grinding wheel is swung around the y
1 axis at an angular velocity w
1 , and the obtained instantaneous equation at time t is converted into the coordinate system s, and then rotated relative to the O
z axis at an angular velocity w
2 to obtain a relative motion. The envelope surface of the sand contour family (ie, the rake face) equation is
 | (3) |
(3) The spherical surface corresponding to the first formula in the equations is
| x *2 +y *2 +z *2 =R 2 | (4) |
The intersection of the spherical surface and the rake face is the cutting edge curve. In order for the edge curve to be an ideal "S" curve, the design variables should be preferred. Design variable is
| X=[X 1 ,X 2 ,X 3 ]=[p,q,R 2 ] | (5) |
Where q=w
2 /w
1 . The specific preferred mathematical model can be found in "Research on Mathematical Models in the Manufacturing of Spherical Milling Cutter" by Shi Pei Lin, Wang Wei, and Tang Yuyong (published in Journal of Mechanical Engineering, No. 5, 1994), which is omitted here.
Figure 2 Principle of flank processing |
4 flank processing principle and trunk model Let the spherical edge curve obtained by equations (3) and (4) be
| r 2 ={x 2 ,y 2 ,z 2 } | (6) |
The ground flank must pass the edge curve and have a sufficient relief angle. The knives processing principle designed for this purpose is shown in Figure 2. In the figure, P
0 is the fixed point on the y-axis (0, y
0 , 0), P
2 is any point on the cutting edge curve, P is the corresponding point on the plane profile curve, and the P-point trajectory is used to ensure the sand contour The surface is ground along a spherical curve. When the P
2 point is perpendicular to the straight busbar and the vertical axis of the grinding wheel axis P
0 P is at point P, only the direction vector
P 2 P can be obtained to obtain the P point coordinate, and then the line P
0 P and y can be obtained. = trajectory of the intersection point
P (
x , y
0 , z) of the
y 0 plane (ie, the master curve). Let the unit vector on the vector
P 2 P be
t = {
,
,
}, the cylindrical grinding wheel radius is R
1 , then there is
 | (7) |
Find the unit vector
t = {
,
,
}, then the P-point trajectory equation is
Then the equation of the straight line P
0 P is
| r p = r p 0 +l( r p - r p 0 )/( r p - r p 0 ) | (9) |
The intersection of the straight line and the plane y=y
0 is the master curve.
Due to space limitations, this article will not list the specific expressions of the above-mentioned main mathematical models. It should be pointed out that the mathematical model given in this section is more concise than the corresponding mathematical model in other literatures, and the corresponding profile curve is easier to manufacture and easy to replace (to meet the needs of processing different specifications of the tool), so it has obvious superiority. Sex. When machining the flank, there is a problem of optimizing the selection of y 0 and R 1 values. The selection principle is to make the tool have a certain back angle and to ensure sufficient strength at the cutting edge. The specific treatment method is omitted here.
5 Conclusion The basic principle and main mathematical model of the non-CNC machining of the ball-end milling cutter introduced in this paper have been successfully verified by the production practice. At present, the ball-end milling cutter series products developed in cooperation with the high-tech park of Harbin Institute of Technology have been put on the market. In addition to guiding the low-cost mass production of ball-end milling cutters, this paper also has reference value for the mass production of other special rotary milling cutters mentioned in "Notes on Two-axis CNC Machining Ball-end Milling Cutter". . However, it should be noted that the content of this paper only reflects the basic framework of the non-CNC machining principle of ball-end milling cutter. If the machining method needs to be realized in the process, the corresponding non-backbone model should be complemented according to other literatures and the framework of this paper. Guide production.
, 
, 
}, the cylindrical grinding wheel radius is R 1 , then there is 
